The world that we perceive is primarily governed by the laws of classical mechanics (think “laws of motion”). Due to thermal energy at room temperatures, often, the effects of the quantum world get concealed. Whereas when we bring down the temperature conditions to the ultracold regime, the quantum world’s impact gets reinforced due to the cancellation of these thermal effects! This enormous decrease in temperatures opens up the path to the new quantum phenomenon, which aids us in understanding the chemical reactions that may otherwise defy explanation. Thus, entering into the ultracold regime renders us with the power to know the unknown.
Why do we need ultra-cold temperatures to observe quantum phenomena?
In 1923, Louis de Broglie, based on Planck’s and Einstein’s, introduced wave-particle duality. He hypothesized a relationship between momentum and wavelength of a particle, λ = h/mv. This relationship can be used to explain the observation of quantum behavior at small scales like for the electron, which has a tiny mass of 9.1×10-31 Kg. Due to its inverse relationship with the wavelength, it has significant wave-like properties. On the other hand, all the macroscopic objects have negligible wave-like properties due to their heavy mass.
The next step up in complexity from electrons is a quantum gas, a system of indistinguishable quantum particles. If we could theoretically label all the particles in a quantum gas, then indistinguishability implies that we can’t identify which particles are different from the rest, i.e., all are identical. While in a labeled classical gas, we can precisely distinguish particles based on their position and momentum. To understand a quantum gas’s properties, instead of just electrons, we now need the whole atom to behave quantum mechanically. For such a system, the atoms’ de Broglie wavelength should be of a length comparable to the spacing between the atoms in low-density gases, i.e., nearly a micron. However, the mass of an atom is almost 10,000 times the mass of an electron. Therefore, the atom’s wavelength is 1/10,000th times the electron’s wavelength.
To increase the de Broglie wavelength of an atom to a micron, we use the relationship between kinetic energy and temperature of the system:
![]()
Substituting p with the de-Broglie wavelength λ = h/p, we get:
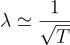
Therefore, to achieve the desired wavelength, we need to cool down the system to microKelvin temperatures. Thus cooling to ultracold temperatures brings down the frantic motion of atoms at room temperatures to a speed even slower than a garden snail. Now that we have achieved a quantum gas, it opens up new exciting opportunities for research.
How Cold is Ultracold?
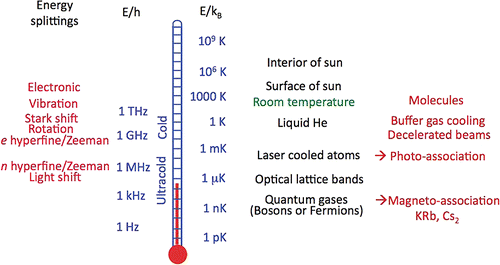
Researchers define cold temperatures to lie between 1mK to a few K, while the ultracold regime is defined below 1mK. Experiments in recent years have achieved temperatures typically of the orders 10 nK to 10 μK. One should realize this is nine orders of magnitude less than the room temperature and six orders lower than the interstellar space temperature. Figure 1 gives an idea about various phenomena associated with different ranges of energy.
How to cool atoms to become ultracold?
The most widely used technique to cool down atoms is Laser Cooling. Now, this might sound counter-intuitive to some people. Usually, due to their directed form of energy, we associate lasers with the heating of objects, such as in Laser welding. But here we are, using them to cool down instead! This is because atoms are altogether a different case. Laser light consists of photons, and when these photons possess the same orientation, frequency, and direction of propagation, they start oscillating in the same phase. This state is extremely ordered in terms of entropy (a thermodynamic measure of the order of the system); thus, lasers are considered extremely cold. When atoms absorb these photons, they emit light of different frequencies. This energy difference causes cooling, which brings down the temperature of the system to the microKelvin range.
Going down further to nanoKelvin scales is done through a process known as Evaporative Cooling. We can understand it with an analogy of a coffee cup. The most energetic molecules leave the surface as steam, thus reducing the coffee molecules’ average energy. This removes the most energetic molecules and cools down the system selectively.
Chemists, however, are more interested in dealing with molecules than atoms. But due to the larger size of molecules, they become less responsive to laser light. Also, the presence of complex energy levels such as the vibrational, rotational, and translational motion of the atoms in the molecule makes it exponentially challenging to cool them.
Unlike atoms, molecules, however, possess a net dipole moment. Similar to how tiny bar magnets get aligned in the direction of an external magnetic field, we can couple and control dipole moments of molecules using electric fields. Interestingly, bringing these molecules down to ultracold temperatures makes it possible to trap and control them by light! This feature of ultracold molecules makes them favorable to develop qubits, which are then combined to form Quantum Computers.
But does Chemistry still take place at ultracold temperatures?
Classically, Arrhenius’ law predicts an exponential decrease in reaction rates with a decrease in temperature.
![]()
where Ea denotes the activation energy, and A is the pre-exponential factor. It also predicts that reaction rates tend to zero when collision energies become vanishingly small in the ultracold regime. Does that imply that reactions cease to exist in the ultracold regime?
To appreciate the chemistry in the ultracold regime, we can consider a model case of the following reaction:
![]()
If H2 is in its ground rotational-vibrational level, the reaction has an energy barrier of about 1.5 kcal mol-1 (~500K) and an exothermicity of about 31.7 kcal mol-1 (1.4eV).
It serves as an essential prototype for hydrogen atom transfer reactions, which is of keen interest to many areas of chemistry, ranging from reactions happening in astrophysical environments (a potential candidate for molecular hydrogen tracer) to reactions taking place in biological environments (tunneling in enzymatic hydride transfer).
Theoretical studies for the above reaction predicted a huge rate constant value of nearly 1.25×10-12 cm3 s-1 at temperatures less than 10mK, which is interestingly of the same order as the rate constant obtained at room temperature [1]. Despite an energy barrier to the reaction, tunneling occurs due to the relatively longer duration of collisions at ultracold temperatures. Quantum tunneling refers to a phenomenon where reactants have a small yet finite probability of penetrating an energy barrier, even if the particle’s total energy is less than the chemical reaction’s activation energy. The larger reaction rate is obtained due to the enhanced tunneling arising from the van Der Waals attraction between the reacting molecules.
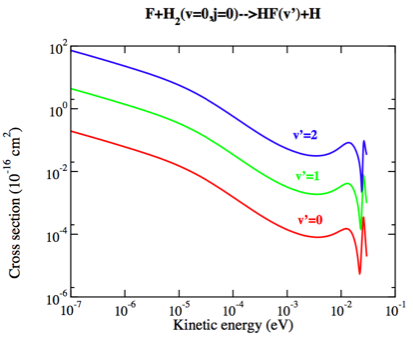
Therefore, we see that quantum threshold effects become increasingly important when going down to temperatures below 1K, where de Broglie wavelength starts becoming comparable to or longer than the distances between the colliding species of a reaction. There has also been evidence of concomitant enhancement of rate constants by degrees of magnitude due to the inherent quantum tunneling contributions in the cold regime (1mK-1K) and higher temperatures (above 10K) [2]. Thus indeed, at ultracold temperatures, chemistry doesn’t stop! Understanding this helps us know about the system’s reaction dynamics and develop quantum-controlled synthesis methods.
In recent years, with modernized equipment development, researchers are achieving new milestones in making molecules orders of magnitudes colder than ever before. In addition to this, the increasing availability of reliable kinetic data obtained at ultra-low temperatures has aided ultra-cold chemistry to see remarkable growth in experimental and theoretical physics and chemistry.
References:
[1]. N. Balakrishnan, A. Dalgarno. Chemistry at ultracold temperatures. Chem Phys Lett, 341 (2001), pp. 652-656
[2]. De Fazio D., Aquilanti V., Cavalli S. Quantum Dynamics and Kinetics of the F + H2 and F + D2 Reactions at Low and Ultra-Low Temperatures. Front. Chem. 2019;7:328.

